Pre-Calculus A 2nd Hour, Fall 2012: fundamental identities

Thursday, November 1, 2012

fundamental identities

Fundamental Identities

You can use SOH CAH TOA if you forget these.

It is also important to know that cosecant is the reciprocal of sine,

Cotangent is the reciprocal of tangent

and Secant is the reciprocal of Cosine.

sin θ=oppositehypotenuse

cos θ=adjacenthypotenuse

tan θ=oppositeadjacent

-Identity is just another word for equivalence, and identities can be used to solve more complex identities and equations.

-To find the trigonometric functions for a given acute angle (theta), you can draw a right triangle that has theta as one of its angles.

Special Angles

$sin30=sin \pi /6=1/2$

$sin45=sin\pi /4=\sqrt{2}/2$

$tan30=sin\pi /6=\sqrt{3}/2$

$tan45=sin\pi /4=1$

$tan60=sin\pi /3=\sqrt{3}$

$sin60=sin\pi /3=\sqrt{3}/2$

$cos30=sin\pi /6=\sqrt{3}/2$

$cos45=sin\pi /4=\sqrt{2}/2$

$cos60=sin\pi /3=1/2$

**sin30=1/2=cos60. This is because 30 and 60 are complementary angles and conjunctions of complementary angles are equal.

there are many groups of trigonometric identities, the first is the reciprocal identity.

displaymath161

the next group is the quotient identity.

there is also the pythagorean identity

In order to Prove the identity, here are some steps you can follow:

1. simplify the more complicated side until it is the same as the other side

2. Try to evaluate the identity in terms of sine and cosine.

3. Use the pythagorean identity as much as you can

4. Use factoring and combining of like terms

some examples include:

verify that:

$sin\theta /cos\theta +cos\theta/sin\theta=csc\theta*sec\theta$

$sin\theta /cos\theta * (sin\theta/sin\theta) + cos\theta/sin\theta *(cos\theta/cos\theta)$

$1 /sin\theta *cos\theta = csc\theta*sec\theta$

$sin^{2}\theta +cos^{2}\theta /sin\theta *cos\theta$

verify that:

$tan\theta /sec\theta-1=sec\theta +1/tan\theta$

$(tan\theta )(sec+1)/(sec\theta -1)(sec\theta +1)$

$(tan\theta )(sec\theta+1)/sec^{2}\theta-1$

$(tan\theta )(sec\theta+1)/tan^{2}\theta$

$sec\theta+1/tan\theta$

Functions on the XY plane

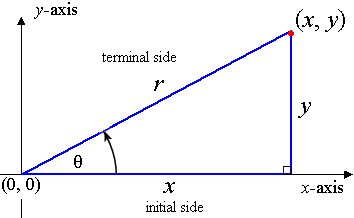
math expression

Standard position:

sin θ=yr

cos θ=xr

tan θ=yx

For further help, watch these videos!

http://www.youtube.com/watch?v=OmJ5fxyXrfg&feature=relmfu

http://www.youtube.com/watch?v=Zktxkfr9zJE

http://www.youtube.com/watch?v=OJz-fOzFbEc

http://www.youtube.com/watch?v=IE0FxGegdMg&feature=relmfu

No comments:

Post a Comment

Subscribe to: Post Comments (Atom)