Composition of Functions

The standard definition for composition of functions is the application of one function to the results of another. The notation used is . Note: The circle in the middle of the composition is NOT a multiplication symbol, it is the symbol for composition notation. Also, the letters "f" and "g" can be substituted for any other letter, they are just used the most often for this type of notation. Another way to say  

is "f composed of g."

Now, for some examples:

Take f(x) = 2x+1 and g(x) = 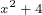. Suppose we want to find .

First we would substitute what g(x) equals which is 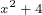 for "x" in the equation for f(x).
This would look like: f(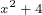) = 2(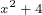) + 1. Now, we can solve the equation.

f(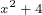) = 2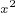 + 8 + 1.         Distribute 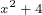 and 2.
f(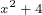) = 2 + 9 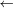               Combine like terms.

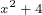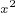Answer: f() = 2 + 9.


What would happen if the composition of the function was ? The answer will be different in this case.

Substitute what f(x) equals which is 2x + 1 for "x" in the equation for g(x).
This would look like: g(2x + 1) =  + 4. Now solve.

g(2x + 1) = (2x + 1)(2x + 1) + 4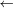             Set up FOIL.
g(2x + 1) =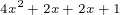  + 4 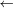       Use the FOIL method to distribute.
g(2x + 1) = 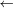                       Combine like terms.

Answer: g(2x + 1) = 



For any other information use this helpful website:
http://www.mathsisfun.com/sets/functions-composition.html


Also, this video shows other examples of how to do composition of a function: